What Is The Volume & Surface Area of a Trapezoidal Prism [All You Need To Know]

A trapezoidal prism is such a prism that the polygons involved are trapezoids. The definition of the prism is a geometric body such that it is formed by two equal and parallel polygons with each other and the rest of their faces are parallelograms.

A prism can have different shapes, which depend not only on the number of sides of the polygon but on the polygon itself.

If the polygons involved in a prism are square, then this is different from a prism that involves rhombuses for example, even though both polygons have the same number of sides. Therefore, it depends on which quadrilateral is involved.

Characteristics of a Trapezoidal Prism

To see the characteristics of a trapezoidal prism you must start by knowing how it is drawn, then what properties the base meets, what the surface area is and finally how its volume is calculated.

1- Drawing a trapezoidal prism

To draw it, it is first necessary to define what a trapezoid is.

A trapezoid is an irregular polygon with four sides (quadrilateral), such that it has only two parallel sides called bases and the distance between its bases is called height.

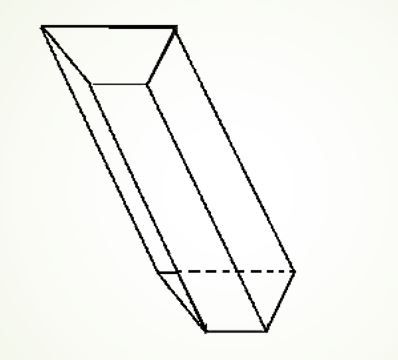
To draw the right trapezoidal prism you start by drawing a trapezoid. Then, a vertical line of length "h" is projected from each vertex and finally, another trapezoid is drawn such that its vertices coincide with the ends of the lines drawn above.

You can also have an oblique trapezoidal prism, whose construction is similar to the previous one, you just have to draw the four lines parallel to each other.

2- Properties of a Trapezoidal Prism 

As said before, the shape of the prism depends on the polygon. In the particular case of the trapeze we can find three different types of bases:

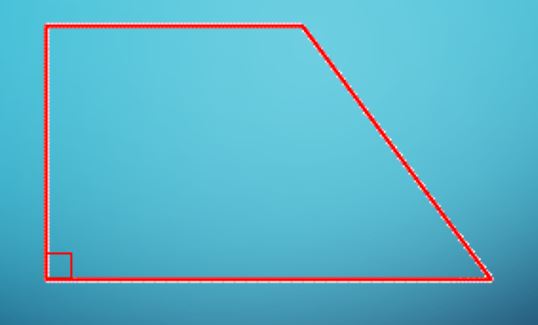
-Rectangular trapezoid: it is that trapezoid such that one of its sides is perpendicular to its parallel sides or that it simply has a right angle.

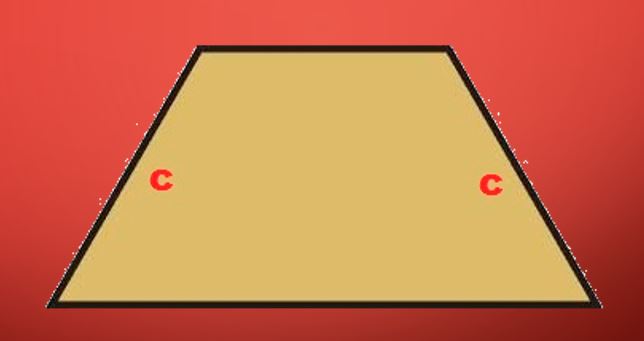
- Isosceles trapezoid: it is a trapezoid such that its non-parallel sides have the same length.

Scalene trapezoid: it is that trapeze that is not isosceles or rectangle; Its four sides have different lengths.

As can be seen according to the type of trapezoid used, the different prism will be obtained.

3- Surface area of a Trapezoidal Prism

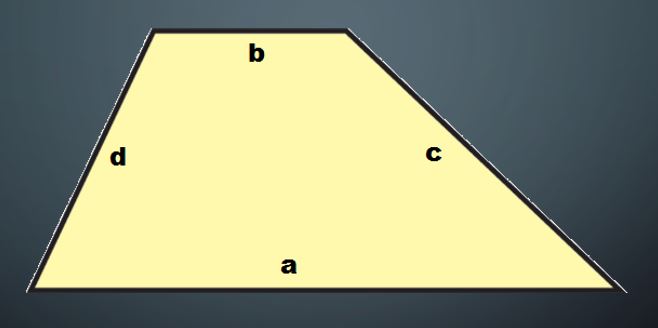
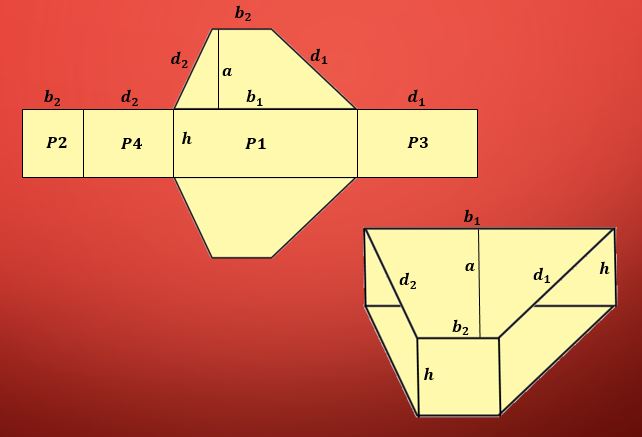
To calculate the surface area of ​​a trapezoidal prism, we need to know the area of ​​the trapezoid and the area of ​​each parallelogram involved.

As can be seen in the previous image, the area involves two trapezoids and four different parallelograms.

The area of ​​a trapezoid is defined as T = (b1 + b2) xa / 2 and the areas of the parallelograms are P1 = hxb1, P2 = hxb2, P3 = hxd1 and P4 = hxd2, where “b1” and “b2” are the bases of the trapeze, "d1" and "d2" the non-parallel sides, "a" is the height of the trapeze and "h" the height of the prism.

Therefore, the surface area of ​​a trapezoidal prism is A = 2T + P1 + P2 + P3 + P4.

4- Volume of a Trapezoidal Prism

Since the volume of a prism is defined as V = (area of ​​the polygon) x (height), it can be concluded that the volume of a trapezoidal prism is V = Txh.

5- Applications

One of the most common objects that have the shape of a trapezoidal prism is a gold ingot or the ramps used in motorcycle racing.

References

1. Clemens, SR, O'Daffer, PG, & Cooney, TJ (1998). Geometry. Pearson Education.
2. Garcia, WF (sf). Spiral 9. Editorial Norma.
3. Itzcovich, H. (2002). The study of figures and geometric bodies: activities for the first years of schooling. Noveduc Books.
4. Landaverde, F. d. (1997). Geometry (reprint ed.). Editorial Progreso.
5. Landaverde, F. d. (1997). Geometry (Reprint ed.). Progress.
6. Schmidt, R. (1993). Descriptive geometry with stereoscopic figures. Reverte
7. Uribe, L., Garcia, G., Leguizamón, C., Samper, C., & Serrano, C. (sf). Alpha 8. Editorial Norma.